Propagation of Radio Waves:

Ground-Wave Propagation and ground effects:

1. Factors Affecting Ground-wave Field Intensity. The ground wave is here considered to be that portion of the radio wave that is affected by the presence of the ground and that would account for all the energy reaching the receiver if it were not for ionospheric waves (sky waves) and tropospheric waves.

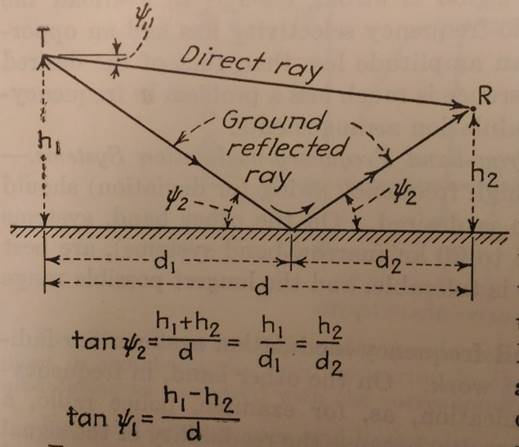
The ground wave can conveniently be divided into two components, a surface wave and a space wave. The surface wave travels along the surface of the earth. The source wave is the result of two component wave as illustrated in Fig.1, namely, a direct wave and a ground-reflected wave. When the transmitting and receiving antennas are both at the earth’s surface, these two components of the space wave are equal in magnitude and opposite in phase, thereby canceling and leaving the surface wave as the only component of the ground wave. This is the case of “ground-wave” transmission of broadcast frequencies. However, as the antennas are raised the amplitude of space wave rapidly increases, and it soon becomes the principal part of the ground wave. This is the condition existing at ultra-high frequencies when the antennas are raised a few wave lengths or more above the earth.

                The magnitude of the ground wave, and of its individual components, the space and surface waves, is influenced by the resistivity and dielectric constant of the earth, the frequency, the height of the transmitting and receiving antennas, the earth, the frequency, the height of the transmitting and receiving antennas, the earth’s curvature, the distance to the transmitter, and the variation of refractive index of earth’s atmosphere with height. The electrical constants of the earth affect the rate of attention of the surface wave, and also the reflection coefficient to which the ground-reflected component of the space wave is subjected. The height of the transmitting and receiving antennas affects the relative amplitudes and phases of space and surface waves, and so influences the resulting field. The curvature of the earth makes it necessary for both the space and surface waves to diffract around the earth in order to reach a distant receiving point that is obscured by the curvature of the earth. The variation of density of the earth’s atmosphere with height, particularly the variation of moisture content, causes the refractive index of the atmosphere to decrease slowly with height above earth. This cause the paths followed by the waves to be slightly curved, instead of straight as show in Fig 1. This curvature is in the same direction as the curvature of the earth’s surface, but is usually somewhat smaller in amount.

2. The Surface Wave. The surface wave is of importance because it represents the whole of the ground wave when both transmitting and receiving antennas are located at the surface of the earth. It accounts, for the daytime coverage of broadcast stations.

Plane Earth Condition (Short Distance) –When the distance from the transmitting antenna is not too great the curvature of the earth’s surface can be neglected. If the heights of transmitting and receiving antennas are low enough that the respective numerical height q₁ and q₂ as calculated by E_q. (14) satisfy the relation (q₁ +q )<0.01, then the surface wave is given by

=A

Where  = field intensity of surface wave in same units as

             d = distance, in same unit of distance as used in

                A= factor taking into account the effect of the earth,

 =a constant determined by the field radiated along the horizontal as discussed below.

                The constant  is the free-space field produced at unit distance from the transmitter in free space with the same antenna currents as are actually present when the antenna is instead near the earth. When the transmitting antenna is vertical, and short compared with   so  that the field radiated is proportional to the cosine of the angle of elevation, then 2=300  millivolts per meter at one km, or 2=186.4  millivolts per meter at one mile, where P is the radiated power in kilowatts. With other vertical antenna arrangements, the value of 2 should be modified by the ratio of the field strength produced by the actual antenna for one kilowatt of power, to the field strength produced by the short vertical antenna.

                The quantity A takes into account the effect of the losses in the earth upon the surface wave, and depends in a relatively complicated way upon the frequency, dielectric constant, and conductivity of the earth, and the actual distance. By making simplifying assumptions that do not introduce appreciable error under the conditions existing in practical radio communication, this reduction factor can be expressed in terms of two parameters, the numerical distance and the phase constant b.

                At large numerical distances (p>10), the factor A is inversely proportional to distance, and has a value approximating 1/2p. Under these conditions the strength of the surface wave is inversely proportional to the square of the distance.